An instructional challenge through problem solving for physics teacher candidates

The study examined a case of two cohorts of pre-service physics teachers from two consecutive academic years in the teacher education program at the University of British Columbia. Each cohort was assigned the same particular problem solving task, that we saw as amenable to a variety of problem solving approaches, with the expectation that the teacher candidates would generate as many approaches as possible, including at least one that drew from mathematics knowledge familiar to the majority of grade 11 and 12 students.

Firstly, the pre-service physics teachers solved the problems individually. Then, they were asked to note similarities and differences as they shared their solutions in groups of three or four. Each group compiled successful problem solving approaches as developed by individual members, which were then presented on overhead transparency sheets to the rest of the class for discussion. The challenge our teacher candidates faced was to generate several problem solving approaches, at least one of which would utilize mathematics knowledge that would be familiar to the majority of grade 11 and 12 high school students. In other words, the problem solving approaches should be derived, as much as possible, from the mathematics knowledge and skills possessed by or familiar to students in grades 11 and 12.

We chose the problem for this assignment based on how challenging and amenable it was to different problem solving approaches. We saw possible solution pathways that ranged from the use of the more advanced to less advanced mathematics knowledge and skills, which in turn was used as a way to evoke and elicit a variety of methodological schema among our pre-service physics teachers. Thus, we used a problem of a slightly more advanced level than is normally found in high school physics in order to provide enough challenge for our teacher candidates, and at the same time generate the level of discussion that illustrated our intended message: teachers need to be sensitive to students' prior knowledge levels. Our teacher candidates recorded their problem solving approaches on transparencies, which along with discussion transcripts, were subsequently used for our in-depth analysis. In this paper, we categorized the solution approaches according to a schema that represented the physics and mathematical knowledge found in each problem solving approach.

The problem

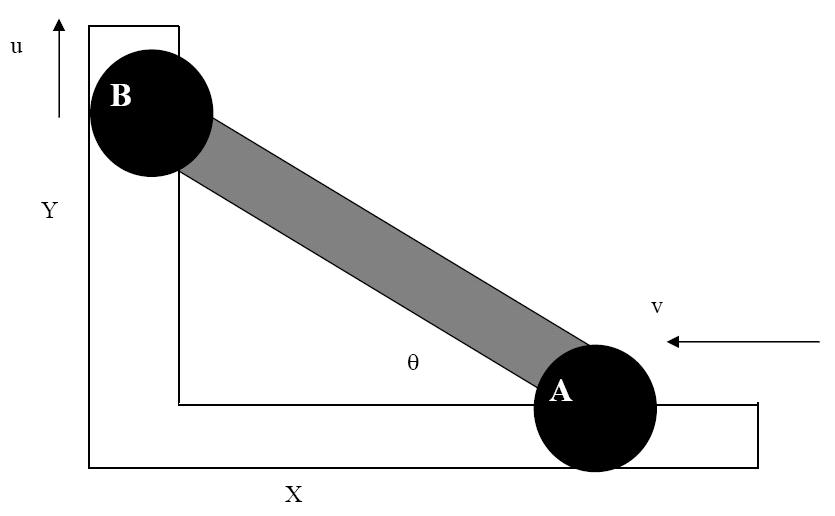
A rigid rod that has length L connects two objects, A and B. The objects slide along perpendicular guide rails as shown in Figure 1 below.

Figure 1. The physics problem

If A slides to the left with a constant speed v, find the speed of B when θ = 60.0 degrees.

Adopted from:
Physics for Scientists and Engineers, 5th Ed
by Serway, R. A & Belchner, R. J. (2000, p.56)